Answer
The region R looks like this:
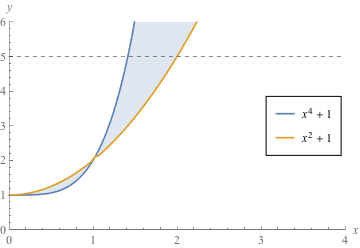
(a) With vertical slices we have to split up the region into three pieces!
The graph y = x4 + 1 intersects the line y = 5 when  . The graph y = x2 + 1 intersects the line y = 5 when x = 2.
. The graph y = x2 + 1 intersects the line y = 5 when x = 2.
1) For 0 < x < 1 the graph of x2 + 1 is on top.
2) For  the graph of y = x4 + 1 is on top.
the graph of y = x4 + 1 is on top.
3) For  the graph of y = 5 is on top.
the graph of y = 5 is on top.
This means there are three pieces whose areas we need to find.
Let's look at the left piece, where 0 ≤ x ≤ 1.
The height of a slice at position x is
(x2 + 1) – (x4 + 1) = x2 – x4.
The area of this slice is
(x2 – x4) Δ x.
The area of the left piece of R is
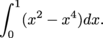
Now for the middle piece, where  .
.
The slice at position x has height
(x4 + 1) – (x2 + 1) = x4 – x2.
This means the area of the slice is
(x4 – x2) Δ x.
The area of the entire middle piece of R is
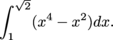
Finally, the right piece, where  .
.
The slice at position x has height
5 – (x2 + 1) = 4 – x2.
The area of this slice is
(4 – x2) Δ x,
and the area of the right piece of R is
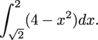
Putting all the integrals together, the area of R is
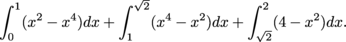
\item With horizontal slices, we need two integrals. The graphs y = x4 + 1 and y = x2 + 1 cross over each other at the point (1,2). So we need to split R into a lower region where 1 ≤ y ≤ 2 and an upper region where 2 ≤ y ≤ 5.
In the lower region, the length of the slice at height y is
(y – 1)1/4 – (y – 1)1/2.
The area of the lower region is
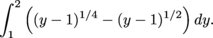
In the upper region, the length of the slice at height y is
(y – 1)1/2 – (y – 1)1/4.
The area of the upper region is

Adding these integrals, we find that the area of R is
