ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
SAT Math: Changing Radicals into a Solvable Quadratic Equation 0 Views
Share It!
Description:
What value(s) of x satisfy the above equation?
Transcript
- 00:00
Yeah Whoa Okay I see T mash bumpers on the
- 00:05
radical problem for you You got a growed to expose
- 00:08
Five is x minus four Question what value of X
- 00:13
satisfy the above equation So what excuse do we have
- 00:17
here All right well this is one radical equation It's
Full Transcript
- 00:20
all about overthrowing modern society And having everyone make a
- 00:23
living is a butter churn Er what can we do
- 00:25
to change this radical into something a little more civilization
- 00:28
friendly Well square both sides of the equation for one
- 00:31
So let's just go do that thing And we've got
- 00:34
then to X minus five is the quantity x minus
- 00:37
four squared What Looks like we have a much less
- 00:39
anti social quadratic on our hands Now multiply out the
- 00:42
term on the right and then gather all the light
- 00:45
terms on one side of equations that gets us Was
- 00:48
that X square than minus Its foreign minus eight Acts
- 00:52
plus 4 square 16 Then we had to explain it's
- 00:54
five over here So let's just get that over here
- 00:57
Two zeros we're gonna add five there gets twenty one
- 00:59
then we're going to subtract two x here so it
- 01:02
gets is already on the ten X ofthe equation is
- 01:04
X squared minus ten x plus twenty one Oh I
- 01:07
see A three and seven Our future Now it's factoring
- 01:10
time So what is it while X minus three and
- 01:12
X minus seven right Well the solutions to this quadratic
- 01:15
equation are X equals three and seven rights Opposite sign
- 01:19
there That was negative SARS or positive which isn't the
- 01:22
correct answer See they would've fooled you with tea there
- 01:25
We don't want the solutions to this quadratic equation We
- 01:28
want the solutions to the radical equation we started with
- 01:32
So we have to plug in three to the original
- 01:34
equation though and no dear things go awry How so
- 01:38
While we get two times three that's six months five
- 01:41
is three months for a square root of one is
- 01:44
negative Once a one equals negative one How can that
- 01:47
be Yeah taking the square root of something I won't
- 01:50
ever give us a negative number So our only solution
- 01:53
is X equal seven So you've got to get rid
- 01:55
of the native one Just keeping a really well
Up Next
In the equation above, if a, b, and c are all constants, what is c?
Related Videos
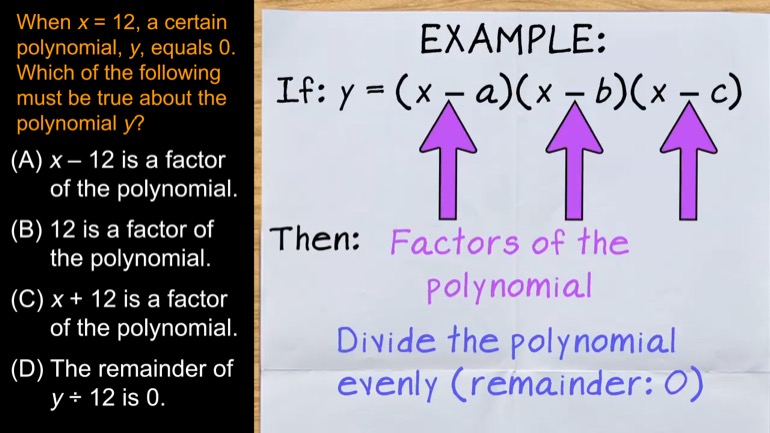
When x = 12, a certain polynomial, y, equals 0. Which of the following must be true about the polynomial y?
SAT Math 1.1 Algebra and Functions. Find an algebraic equation to correspond with the data.
SAT Math 1.1 Geometry and Measurement. What is the circumference of the circle?
SAT Math 1.1 Numbers and Operations. How many combinations of beverage and cereal can be made?