Think you’ve got your head wrapped around Derivatives? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. Find the slope of the secant line between the two points shown on the graph of f(x) = cos(x):

1
-π

Q. Let f(x) = x2 – 1. Find the slope of the secant line between the points (-1, f(-1)) and (2, f(2)).
1
3
0.75
4
Q. Which picture most closely resembles the limit definition of the derivative of f at a?

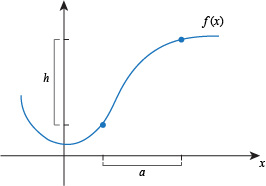
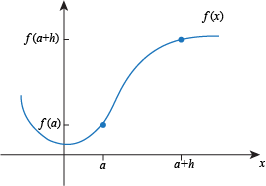
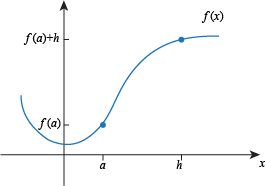
Q. The derivative of f(x) = |x – 1| at x = 1 is
0
1
-1
undefined
Q. Let y = f(x) and assume f ' (a) exists. Which of the following quantities must equal f ' (a)?
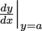
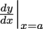
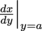
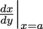
Q. If x is measured in cookies, y is measured in glasses of soda, and z is measured in slices of cake, then  is measured in
is measured in
 is measured in
is measured in cookies per glass of soda
glasses of soda per slice of cake
slices of cake per glass of soda
glasses of soda per cookies
Q. Use the limit definition to find the derivative of f(x) = x2 + x at x = 2.
5
5 + h
2x + 1
undefined
Q. Use the following table to estimate the derivative of f at x = 4.

0.248
0.25
4
4.04
Q. If f(1) = 12 peacocks and f(5) = 36 peacocks, find the average rate of change of f with respect to x on the interval from 1 to 5. The value of x is measured in chocolate bars.
6 peacocks per chocolate bar
6 chocolate bars per peacock
4 peacocks per chocolate bar
4 chocolate bars per peacock
Q. Let f be a function. Which of the following statements is true?
"Average rate of change" and "instantaneous rate of change" mean the same thing.
The average rate of change of f on an interval is the same thing as the secant line on that interval.
The instantaneous rate of change of f on an interval is the same thing as the derivative of f on that interval.
The slope of f at a is the same thing as the derivative of f at a.